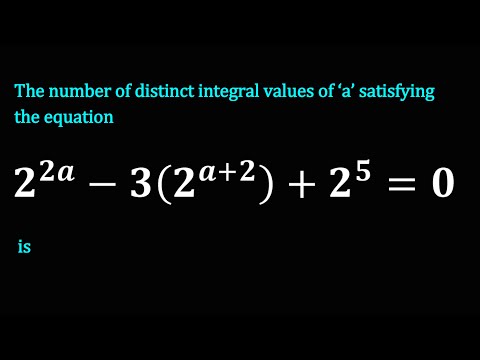
The number of distinct integral values of ‘a’ satisfying the equation 2^2a-3(2^(a+2) )+2^5=0 is
quadratic equation, Impetus gurukul
#quadratic_equation
@Impetus Gurukul
--
To buy complete Course please Visit–
https://www.impetusgurukul.com or contact on 7047832474
join Impetus Gurukul live classes via the official Website:
https://live.impetusgurukul.com
--
Algebra Playlist:
Permutations & Combinations:
https://www.youtube.com/playlist?list=PLPuihxHuENxamdZdXfn0rSBZuHGwusVen
Matrices:
https://www.youtube.com/playlist?list=PLPuihxHuENxZm6YucxIwAm3xcdhJ2bhyO
Determinants:
https://www.youtube.com/playlist?list=PLPuihxHuENxYgOYWpJczv8mnYQYSTPY4q
Binomial Theorem:
https://www.youtube.com/playlist?list=PLPuihxHuENxY-Ii_c2Licb7eG0qriW7PO
Progression ( A.P,G.P, H.P & Special Series):
https://www.youtube.com/playlist?list=PLPuihxHuENxaB7BCOHo9VW_yCvpRz1ZWT
Quadratic equation & Inequations:
https://www.youtube.com/playlist?list=PLPuihxHuENxag_9N2bMJENRKjLaJfY8M-
Set & Relations:
https://www.youtube.com/playlist?list=PLPuihxHuENxabANGYLyuVET_pDCXTrlOD
Complex Number:
https://www.youtube.com/playlist?list=PLPuihxHuENxYbBeNtHXGrBNSFPFNPnoZV
--
Our Social links
Telegram: https://t.me/impetusgurukul
Facebook: https://www.facebook.com/impetusgurukul
LinkedIn: https://www.linkedin.com/in/dr-sharad...
twitter: https://twitter.com/ImpetusSharad
---
Quadratic Equations
A quadratic equation in the variable x is an equation of the form ax2 + bx + c = 0, where
a, b, c are real numbers, a 0.
In fact, any equation of the form p(x) = 0, where p(x) is a polynomial of degree
2, is a quadratic equation. But when we write the terms of p(x) in descending order of
their degrees, then we get the standard form of the equation. That is, ax2 + bx + c = 0,
a 0 is called the standard form of a quadratic equation.
In general, a real number is called a root of the quadratic equation
ax2 + bx + c = 0, a 0 if a 2 + b+ c = 0. We also say that x = is a solution of
the quadratic equation, or that satisfies the quadratic equation. Note that the
zeroes of the quadratic polynomial ax2 + bx + c and the roots of the quadratic
equation ax2 + bx + c = 0 are the same.
Quadratic Formula = [-b ± √(b² - 4ac)]/2a
Important Quadratic Equation Formulas
The following list of important formulas is helpful to solve quadratic equations.
• The standard form of a quadratic equation is ax2 + bx + c = 0
• The discriminant of the quadratic equation is D = b2 - 4ac
• For D ‘Greater than’ 0 the roots are real and distinct.
• For D = 0 the roots are real and equal.
• For D ‘Less than’ 0 the roots do not exist, or the roots are imaginary.
• The formula to find the roots of the quadratic equation is x = −b±√b2−4ac2a−b±b2−4ac2a.
• The sum of the roots of a quadratic equation is α + β = -b/a = - Coefficient of x/ Coefficient of x2.
• The product of the Root of the quadratic equation is αβ = c/a = Constant term/ Coefficient of x2
• The quadratic equation having roots α, β, is x2 - (α + β)x + αβ = 0.
• The condition for the quadratic equations a1x2+b1x+c1=0a1x2+b1x+c1=0, and a2x2+b2x+c2=0a2x2+b2x+c2=0 having the same roots is (a1b2−a2b1)(b1c2−b2c1)(a1b2−a2b1)(b1c2−b2c1) = (a2c1−a1c2)2(a2c1−a1c2)2.
• For positive values of a (a ‘Greater than’ 0), the quadratic expression f(x) = ax2 + bx + c has a minimum value at x = -b/2a.
• For negative value of a (a ‘Less than’ 0), the quadratic expression f(x) = ax2 + bx + c has a maximum value at x = -b/2a.
• For a ‘Greater than’ 0, the range of the quadratic equation ax2 + bx + c = 0 is [b2 - 4ac/4a, ∞)
• For a ‘Less than’ 0, the range of the quadratic equation ax2 + bx + c = 0 is : (∞, -(b2 - 4ac)/4a]
---
+quadratic equation,Impetus gurukul,Relationship Between Coefficients and Roots of Quadratic Equation,Quadratic Equation solutions,JEE previous year's question's solution,Quadratic Equation Formulas,Tricks for Solving Quadratic equation,solve quadratic equation,Quadratic equation roots formula,Sum and product of roots of quadratic equation,nda quadratic equation,NIMCET quadratic equation,jee quadratic equation
0 Comments